Venous systemic model

Mathematical model > Vascular model > Venous systemic model

The system of correlations (7) - (11) is concretized conformably to systemic veins (VL compartment).

Venous systemic pressure P_VL is a pressure in the opening of right atrium, that is a part of venous systemic compartment (VL). This pressure is determined as a sum of a vascular component (7), and a component P_VL0 , defined by atrium activity:

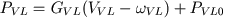(33)

In the formula (33) volumetric elasticity G_VL describes rigidity properties of venous walls, ω_VL - stressless volume in this section of vascular system. Values of volumetric elasticity and stressless volume decribe venous tone. In our model this value G_VL linearly depends on neurohumoral factor γ, and the value ω_VLis a parameter

(34)

The formula (34) describes the increase of venous wall elasticity under the increase of neurohumoral input.

For blood volume V_VL algebraical equation of volumetric balance is given:

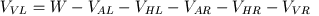(35)

where W - total blood volume, other volumes are determined by differential equations (9).

Blood flow from right atrium to right ventricle through tricupsid valve is determined by this formula variant (21):

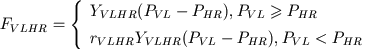(36)

The conductivity of this section of vascular model is evaluated with the equation:

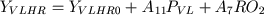(37)

The member A₆P_VL in the equation (37) evaluates the dependence of venous conductivity from venous pressure: pressure increase leads to an expansion of venous lumen and conductivity increase. The member A₇RO₂ expresses a well-known fact of venous pump function (the function of working skeleton muscles). Working muscles are an addition factor, that accelerates venous blood flow.

Mathematical model > Vascular model > Venous systemic model

References on the topic:

Mathematical model > Vascular model> Venous pulmonary model